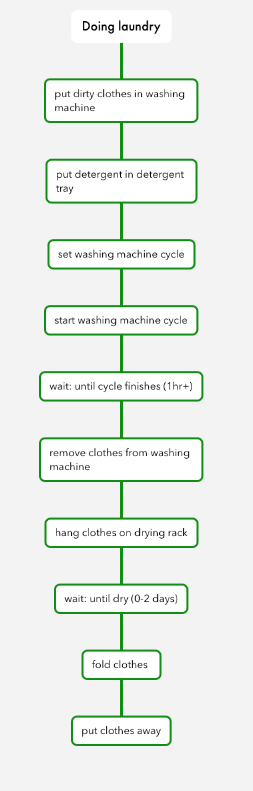
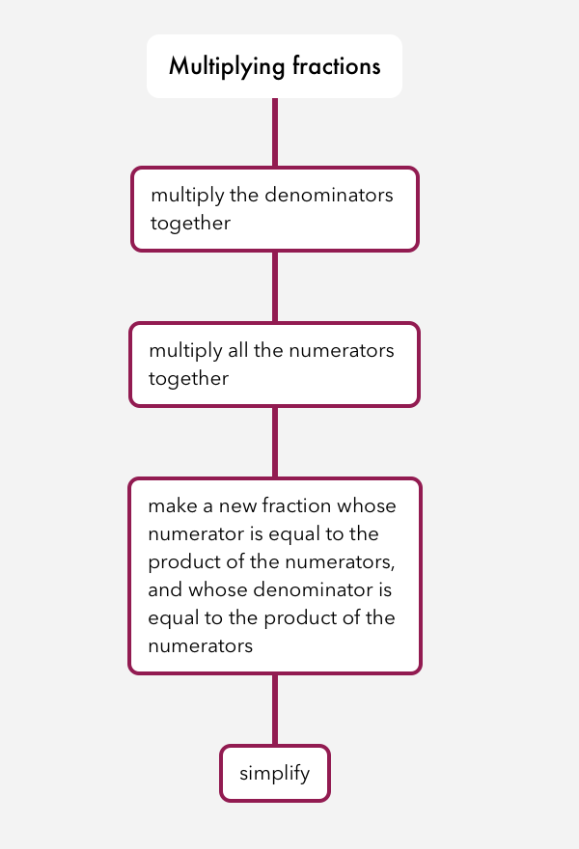
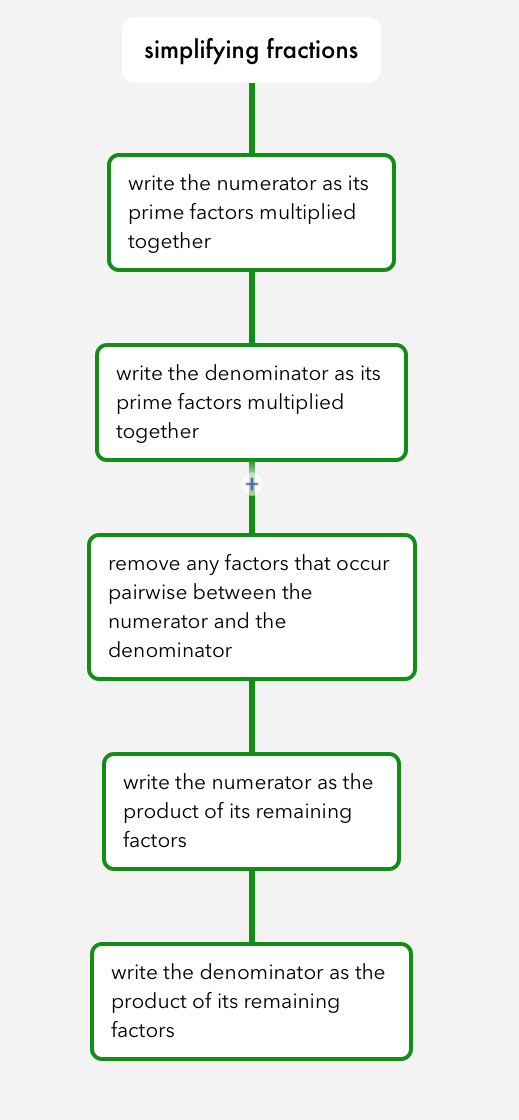
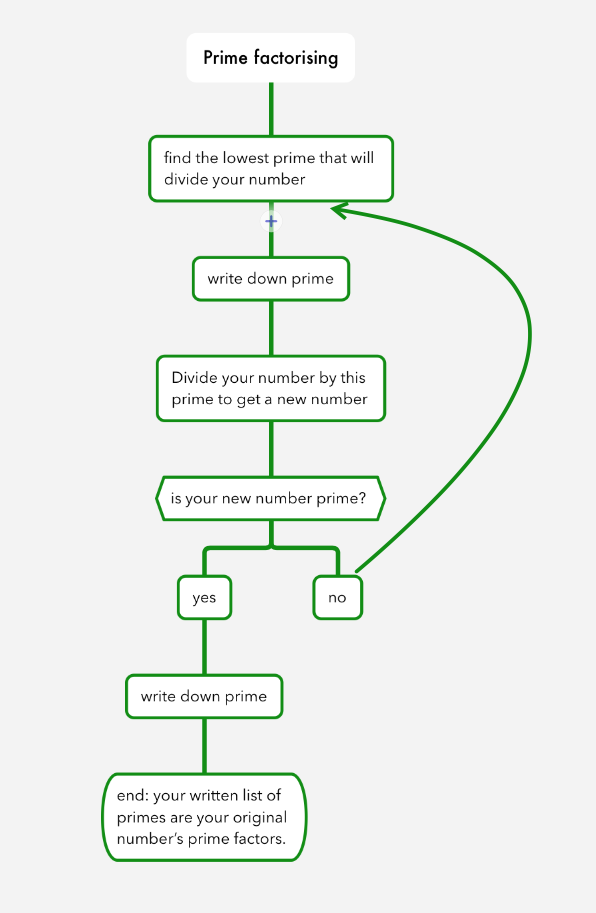
OK how about another flowchart for a household task and another one for math. 5-20min each
Assignment: Write 15+ minutes per day. Subject matter is up to you. I just want to get you more comfortable with writing.
You can do private journaling, you can write thoughts on intellectual issues, you can write todo lists to organize your days, you can write commentary on TV and YouTube, you can write suggestions for your workplace (no need to tell your workplace), you can write notes on things you learned, you can write explanations of how to do things, whatever.
Try not to skip more than 1 or 2 days per week. Keep track of what days you write on and maybe time and word count. But don’t try to make your time or word count go up; big numbers aren’t the goal here.
Share a write every week or two. It’s fine if most of it is private but try to write something shareable sometimes. You can share more if you want to.
Also I think you were or are doing something like this already. Let me know what you’ve been doing and how it’s been going.
How about a flowchart for simplifying fractions.
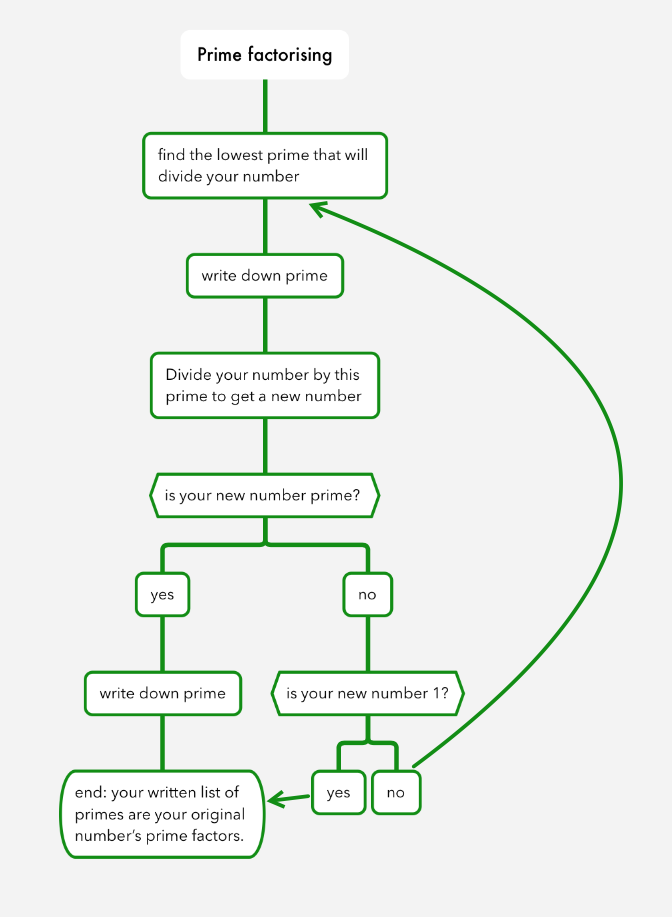
Cool and a flowchart for prime factorizing
I realised that my first prime factorising flowchart couldn’t handle primes. I updated it so that if you start with a prime it’ll give you a list of one prime factor, itself, and then end. Here is an update.
Cool okay. I was writing semi-regularly. I used the Daily Note function in Obsidian to write down thoughts I had during the day or notes on what I was reading or plans I was making or things to remember or brainstorming. Kind of like a memory aid/note pad idea thing. Some days I would end up writing over 1000 words. I did this for about a month or two before we started doing async tutoring, and I haven’t really done any since. I write the occasional paragraph to experiment with getting an idea down still. I do some tutoring journalling to keep track of what I’ve covered per week.
I started again today and wrote ~700 words in ~19mins.
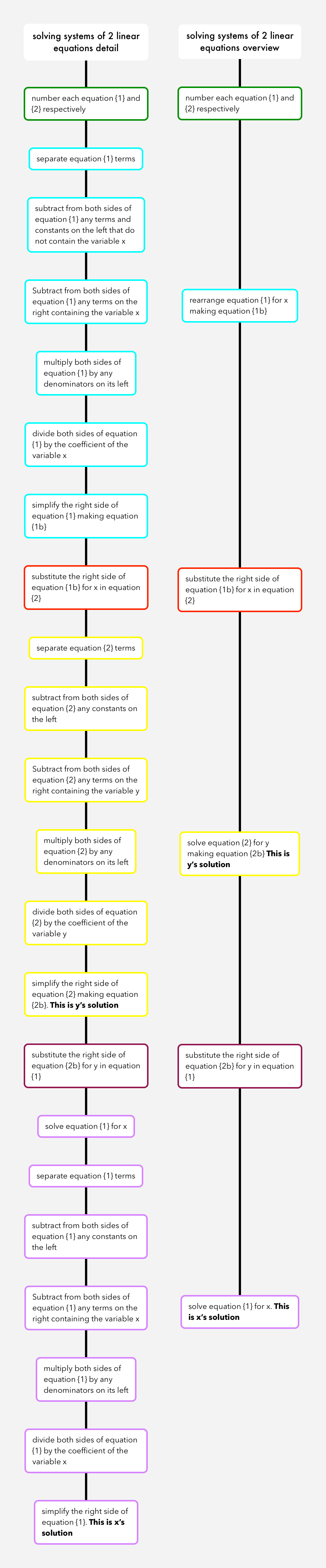
ok do you think you would be able to make a flowchart for solving systems of 2 linear equations now? if so, go ahead, 1h max. if not, what are you thinking you’d have trouble with?
feel free to study and practice those equations more first if desired.
I think so. I’m happy to give it a go to find out.
I somehow didn’t start my stopwatch or take note of the time so I’m not sure exactly how long this took to do. I would estimate ~30mins.
I did two flowcharts. They are the same but the one on the left has more detail and the one on the right has a summary nodes for multiple steps. I color coded them so you can see what nodes correspond between the flow charts. I just realised that I could’ve made a single horizontal tree, numbered the summary nodes, and had the details as numbered children of the summary nodes. Anyway this should do.
try a few problems with your flowchart
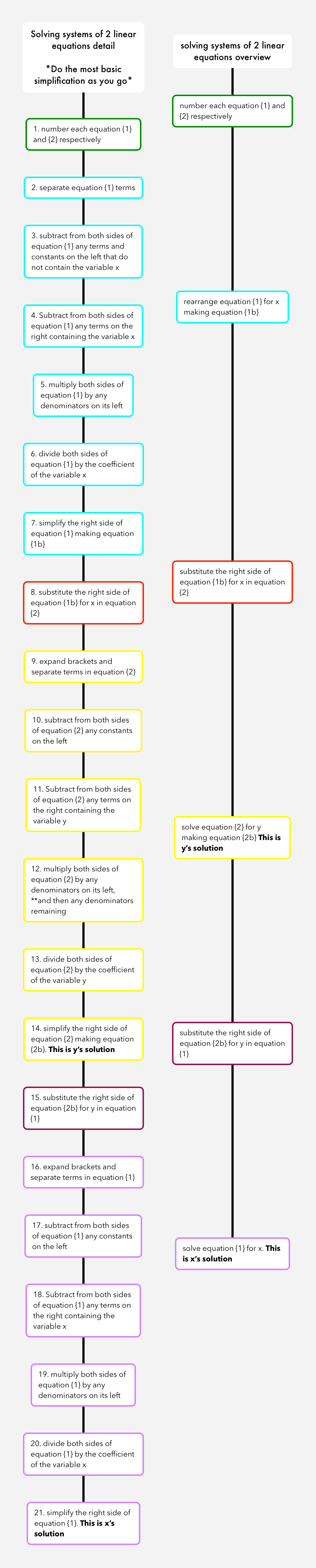
I made some slight changes otherwise for all problems (3) I tried the flowchart gave the correct answers. I numbered all the steps for reference.
Problem: Simplifying as you go or no?
Solution: Added clarification in title node to do the most basic simplification as you go, so that that is assumed throughout.
Problem: Did an problem where after clearing the denominators on the left in step 12 I still had a term with a denominator because a fraction was part of the expression substituted for x in step 8. x was a numerator in the equation.
Solution: I added a further/sub step to 12 to then multiply by any remaining denominators.
Problem: Expanding brackets after substitution steps.
Solution:In steps 9 and 16 after substitution I added ‘expand brackets’.
(I also removed a node from the detail flowchart that was a summary node and there by mistake.)
Here is some working for a problem. I number the steps and put ‘NE’ where a step has no effect. I thought it was cool that the flowchart did some steps differently than I would intuitively but worked anyway.
continued
OK cool, let’s try some word problems, similar to what Eternity has been doing, using my 3 section method.
In the third section, put calculations. (But don’t do that yet. Stop after two sections.)
Should I ignore the instruction in the parentheses you gave eternity?
Either way is OK.
These problems are from here
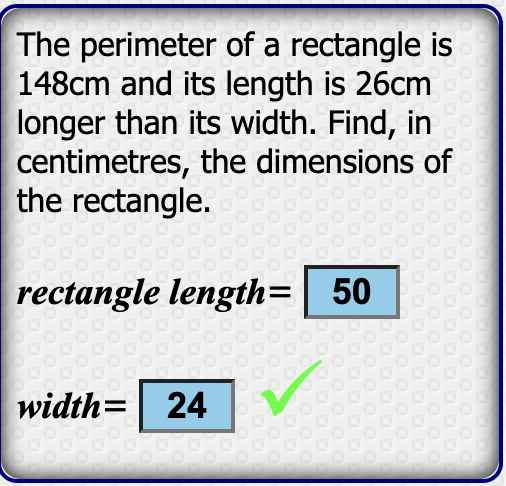
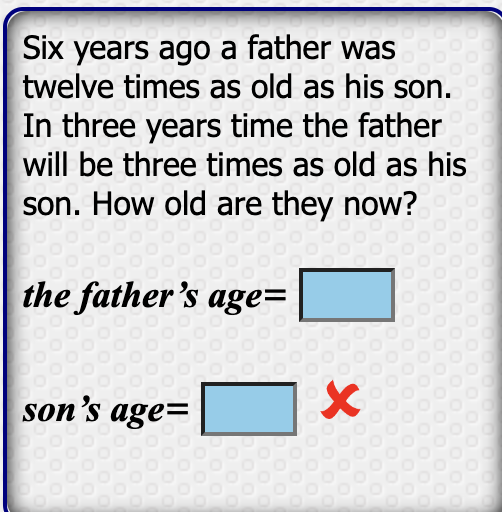
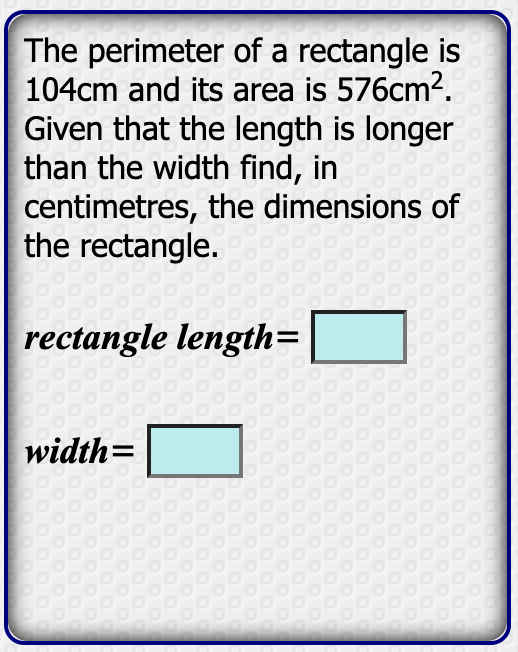
I got stuck on this:
But while typing this post I had an idea, and that worked. I think part of my mistake was not thinking of the the father’s and son’s age as their ages now. So f = fathers age now and s = sons age now.
I’d suggest writing the perimeter equation but you got it anyway. Your second try on the age one is good. Try more problems.
This is from the same site as above which describes these problems as ‘simultaneous equations’ and not systems of linear equations. I’ve noticed some UK/US math terminology differences so I thought they might be synonymous, but I’m not sure they are. Am I right that this problem is not representable as a system of linear equations or am I just missing something? (My equations give a term to the n^2 power)